Have you ever wondered how ancient civilizations made sense of the world around them? For the Maya, mathematics was more than just numbers; it was a vital part of their culture. The Maya civilization, thriving in Mesoamerica from around 2000 BC, achieved incredible feats in architecture, astronomy, and agriculture, all rooted in their advanced mathematical knowledge. They developed a sophisticated number system and understood concepts like zero long before many other cultures. Join us as we explore the fascinating world of Mayan mathematics and uncover the achievements that set this civilization apart. You won’t want to miss it!
Historical Context of Mayan Mathematics
The Maya civilization flourished in Mesoamerica from around 2000 BC until the Spanish conquest in the 16th century. During this time, the Maya developed a rich cultural and intellectual heritage. Mathematics played a crucial role in their achievements, influencing various aspects of daily life, including trade, agriculture, and astronomy.
The Maya relied heavily on mathematics for their intricate calendar systems. They used a vigesimal (base-20) number system, which was essential for tracking time and astronomical events. This system allowed them to create accurate calendars, such as the Tzolk’in and Haab’, which guided agricultural practices and religious ceremonies.
Additionally, mathematics was integral to their architectural endeavors. The precise measurements and geometric principles applied in constructing their impressive pyramids and temples showcased their advanced mathematical understanding.
In daily life, mathematics was essential for trade and commerce, helping the Maya manage resources and calculate quantities. Overall, mathematics was not merely an abstract concept; it was interwoven into the fabric of Mayan society, reflecting their intellectual sophistication and cultural achievements.
The Mayan Number System: Mayan Mathematics
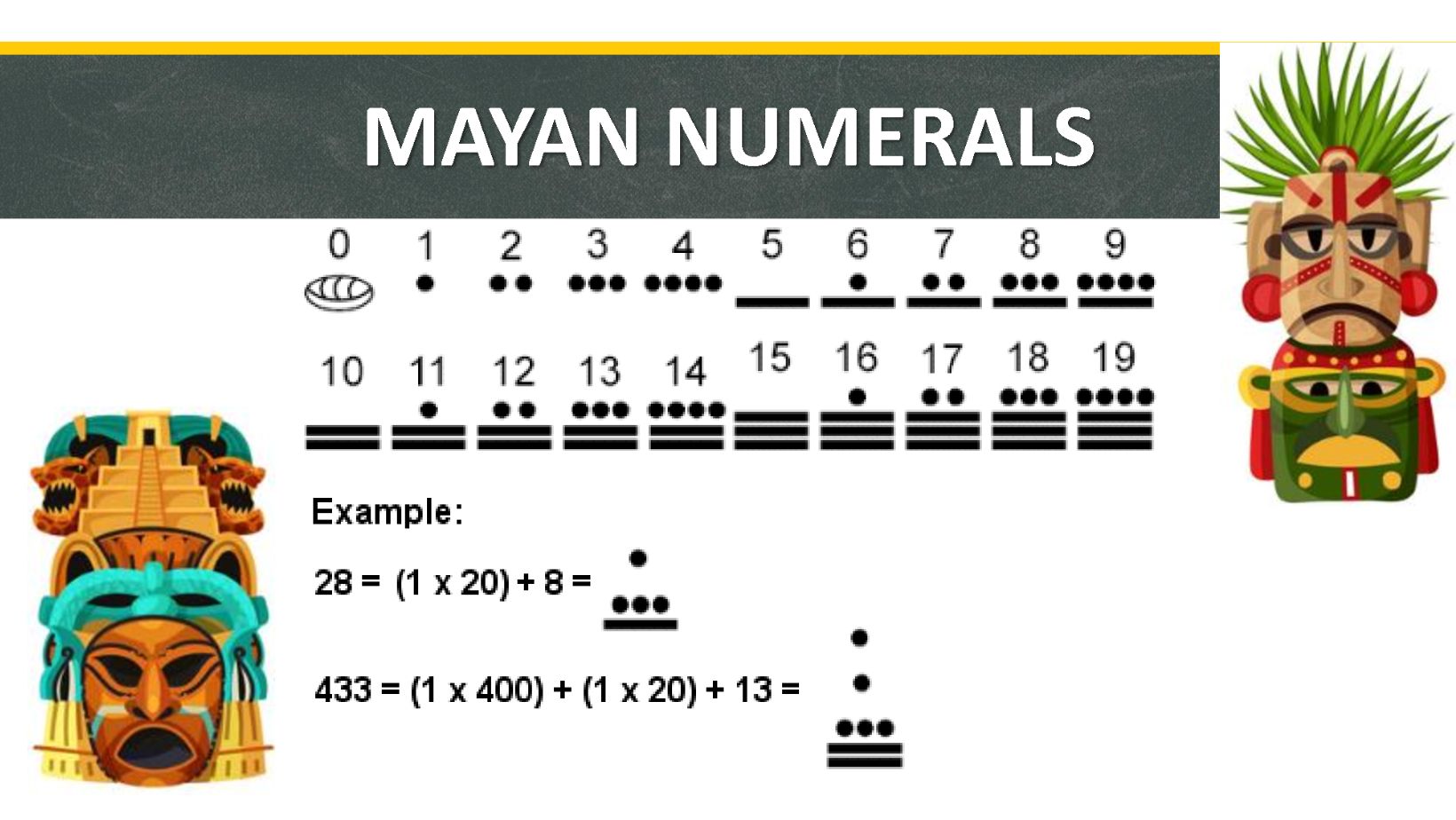
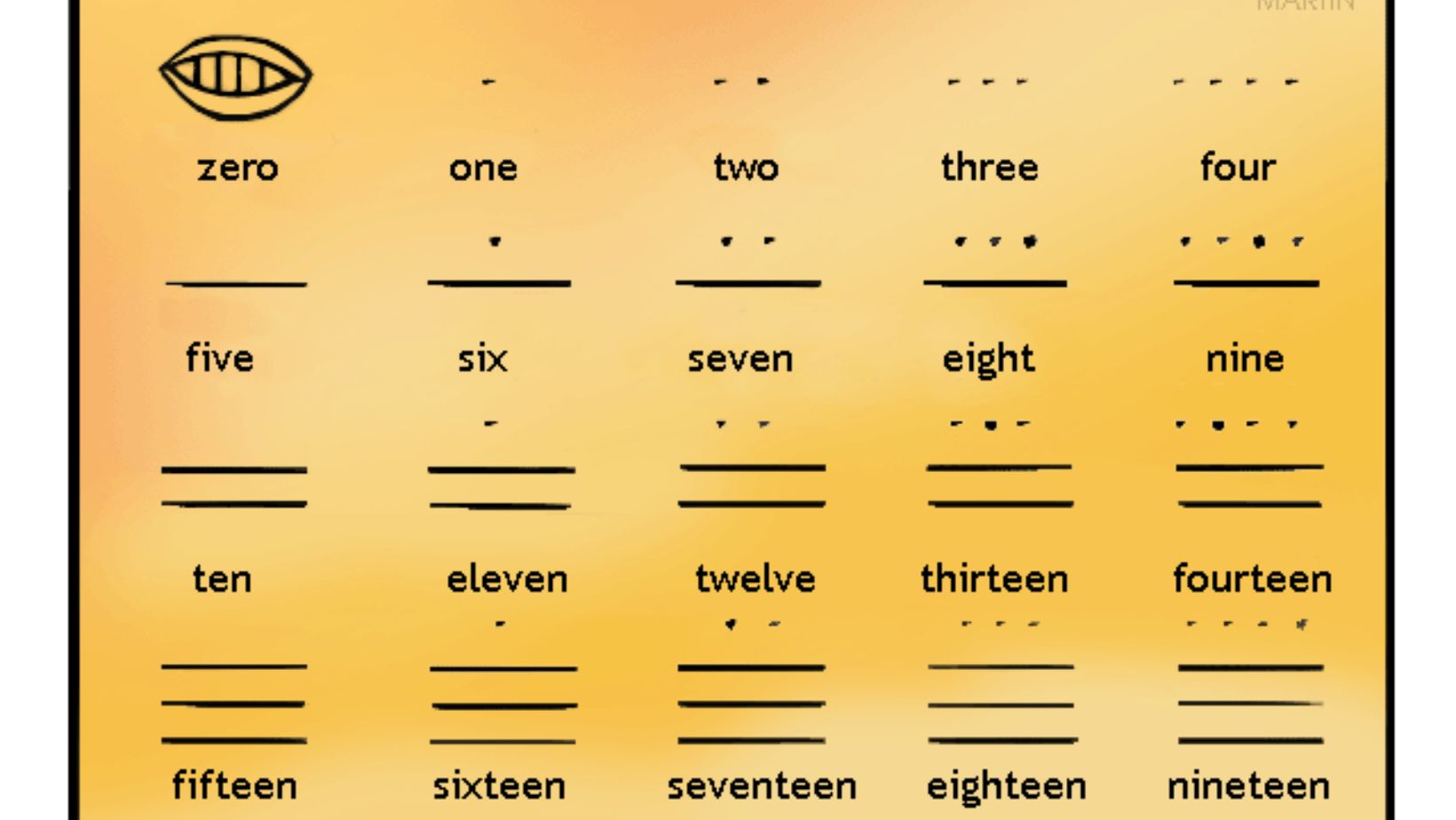
The Mayan number system is fascinating and unique. It is a vigesimal system, meaning it is based on the number 20. This system is different from our decimal (base-10) system. In the Mayan culture, counting started from one and moved up to twenty, then continued with multiples of twenty.
The Maya used a combination of dots and bars to represent numbers. A single dot stood for one, while a horizontal bar represented five. For example, three dots represented the number three, and two bars represented ten. The Maya also used a shell symbol to denote zero, a concept that was revolutionary for its time.
Numbers were written vertically in stacks. The bottom layer represented the lowest place value, similar to how we write numbers today. The higher the layer, the greater the value, reflecting their understanding of place value. This system allowed them to express large numbers efficiently, which was crucial for their astronomical calculations and record-keeping. Overall, the Mayan number system showcases their mathematical sophistication and innovation.
Mathematical Operations: Mayan Mathematics
The Maya used straightforward techniques for addition and subtraction based on their base-20 number system. Addition involved combining dots and bars, with any group of five dots replaced by a bar and groups of four bars replaced by a single dot in the next higher place. Subtraction was the reverse, where bars and dots were removed from numbers, adjusting place values as needed. These methods made calculations accessible and efficient, even for large numbers.
For multiplication, the Maya used a repeated addition approach since they did not have a separate multiplication symbol. They broke down numbers into sums of smaller, manageable parts, adding them step-by-step. This method, while time-consuming, allowed them to solve multiplication problems with accuracy. It was especially useful for calculating areas and resources.
Division was more complex and relied on repeated subtraction, similar to how they handled multiplication by addition. Dividing larger numbers required breaking them into smaller components and then repeatedly subtracting to reach the final quotient. This process was labor-intensive but demonstrated their understanding of mathematical concepts and resource management. The Maya’s methods in these operations reveal a practical, methodical approach to mathematics, suited to their needs in trade, construction, and calendar calculations.
The Concept of Zero: Mayan Mathematics
The Maya were one of the earliest civilizations to develop and use the concept of zero. This concept was critical in their mathematical system, helping them create more accurate calculations and organize place values. Represented by a shell symbol, zero allowed the Maya to perform complex arithmetic and was essential for their calendar systems. Their use of zero stands as one of their most significant mathematical achievements.
Zero played a crucial role in place value, making it possible to distinguish numbers like 20, 200, or 2,000. In the Mayan vigesimal (base-20) system, zero functioned similarly to how we use it today, helping in calculating large numbers accurately. This understanding enabled them to manage intricate calendar cycles and conduct advanced astronomical observations. By incorporating zero, they could track time more precisely than many contemporary societies.
The Maya likely developed zero independently, possibly inspired by the cyclical patterns they observed in nature and time. Their understanding of zero was so advanced that it rivaled mathematical innovations in ancient India, where zero was also independently conceptualized. This mastery of zero highlights the intellectual depth of Mayan society, reflecting their innovation and profound influence on later mathematical developments.
Calendar Systems and Mayan Mathematics
The Maya developed two main calendar systems: the Tzolk’in and the Haab’. The Tzolk’in is a 260-day ritual calendar used for religious events and ceremonies, while the Haab’ is a 365-day solar calendar that aligns with the seasonal year. Together, these calendars formed a “Calendar Round” cycle, lasting 52 years before repeating, which structured religious and agricultural activities. The Maya used precise mathematical calculations to synchronize these cycles, integrating both practical and spiritual life.
Mathematics played a central role in managing these complex time cycles. The Maya tracked days, months, and years by using their base-20 number system, allowing them to calculate large time spans with ease. This helped them align agricultural practices, like planting and harvesting, with seasonal changes. Additionally, they relied on their understanding of mathematical operations to coordinate events, ensuring the Calendar Round stayed accurate.
Astronomy was also deeply connected to their calendar system. The Maya used mathematics to predict solar and lunar cycles, eclipses, and the movements of planets like Venus. These observations allowed them to make advanced predictions, which were crucial for both daily life and religious events. Through their calendars, the Maya demonstrated a profound understanding of time, mathematics, and the cosmos.
Astronomy and Mayan Mathematics
The Maya were highly skilled astronomers who used mathematics to track and predict celestial events. By applying their base-20 number system, they calculated the cycles of the sun, moon, and planets with remarkable precision. Their understanding of numbers and cycles allowed them to track solar and lunar eclipses and even predict the movements of Venus. These predictions were so accurate that they closely matched modern astronomical calculations.
One of their notable achievements was the “Venus Table” found in the Dresden Codex. This record detailed the 584-day cycle of Venus, which the Maya tracked to align important religious events with the planet’s visibility. They viewed Venus as a powerful celestial force, and its cycles influenced military and religious decisions. Their observations of Venus were among the most advanced in the ancient world.
The Maya also built observatories, like the one at Chichen Itza, to study the sky. They aligned their buildings with celestial events, showcasing their knowledge of astronomy and geometry. By combining astronomy with mathematics, the Maya developed a complex understanding of the universe. This knowledge reinforced their belief systems and guided important decisions, blending science with spirituality.
Architecture and Geometry: Mayan Mathematics
The Maya applied mathematics and geometry extensively in their architecture, creating structures that were both functional and symbolic. They used precise measurements and calculations to align buildings with celestial events, such as the equinoxes and solstices. This required a deep understanding of angles, symmetry, and spatial planning. Mathematics allowed them to construct buildings that not only stood the test of time but also held religious and astronomical significance.
One prominent example of geometry in Mayan architecture is the pyramid at Chichen Itza, known as El Castillo. This structure is carefully aligned to create a shadow resembling a serpent on the equinox, a result of exact geometric calculations. The Maya used steps, angles, and heights to create this effect, demonstrating their mastery over architectural design. The pyramid’s nine terraces and 365 steps also reflect the solar year, integrating mathematical concepts into its very structure.
Another example is the layout of the ancient city of Tikal, where buildings are arranged in geometric patterns. These designs facilitated both daily activities and ceremonial practices, showing the Maya’s skill in urban planning. Through their architecture, the Maya expressed their knowledge of geometry, creating structures that aligned with their worldview and cosmology.
Education and Knowledge Transmission
In Mayan society, education in mathematics was essential for those involved in astronomy, calendar-making, and temple construction. Mathematical knowledge was often passed down through a select group of educated scribes, priests, and nobles. These individuals learned mathematics as part of a broader education in astronomy, religion, and governance. This specialized learning ensured that crucial knowledge stayed within the elite class, enabling precise timekeeping and ritual planning.
The Maya preserved mathematical knowledge through codices, which were folded books made from bark paper. These codices contained detailed calculations, calendars, and astronomical tables, safeguarding mathematical principles for future generations. The Dresden Codex, one of the few surviving Mayan manuscripts, includes sophisticated records of cycles for celestial bodies like Venus and the moon. These preserved writings are a testament to the importance the Maya placed on maintaining their knowledge.
Education likely involved practical exercises in counting and using their base-20 number system, especially for those involved in trade or construction. Codices and inscriptions on stelae and monuments helped reinforce this learning, ensuring accuracy in their complex calculations. Through these methods, the Maya effectively preserved and transmitted their mathematical knowledge, leaving a lasting legacy in the historical record.
Legacy and Influence on Modern Mathematics

Mayan mathematics left a lasting legacy that influenced both their own civilization and later cultures. Their advanced understanding of zero, place value, and calendar systems set a high standard in mathematical thought. Although Mayan mathematical concepts did not directly influence other ancient civilizations, their achievements are now recognized as groundbreaking in mathematical history. The rediscovery of these principles highlights the Maya’s unique contribution to the global history of mathematics.
Today, Mayan mathematics is valued for its sophisticated number system and astronomical calculations. Modern archaeologists and mathematicians continue to study their codices and monuments to understand these ancient techniques. The Mayan concept of zero, developed independently, mirrors ideas that only emerged in other parts of the world centuries later. This innovation is especially significant, as zero is fundamental in modern mathematics, computing, and science.
The relevance of Mayan achievements also lies in their precise calendar systems, which still intrigue researchers today. Their ability to track time and celestial cycles reveals an impressive grasp of mathematical cycles. By preserving and sharing these discoveries, scholars honor the legacy of the Maya, recognizing their contributions to math and their lasting influence on our understanding of ancient knowledge.
Conclusion: Mayan Mathematics
Mayan mathematics stands as a testament to the ingenuity and intellectual depth of the Maya civilization. Their innovations in the use of zero, a base-20 system, and precise calendar calculations showcase their advanced understanding of math and time. These achievements not only supported their society but also contributed to human knowledge, influencing how we view ancient mathematics today. The enduring legacy of Mayan mathematical accomplishments continues to inspire modern scholars, highlighting the Maya’s role as pioneers in mathematical thought and astronomical observation. Through their work, they left an indelible mark on history and the study of mathematics.
FAQs
How did the Maya use math in their daily lives?
The Maya applied math in many practical ways, from trade and commerce to architecture and agriculture. Math helped them calculate trade quantities, build precise structures, and track agricultural cycles, ensuring their society was efficient and organized.
Did the Maya use fractions or decimals in their calculations?
No, the Maya did not use fractions or decimals as we do. Instead, they relied on their base-20 system to express large and small quantities, using place values and the concept of zero to simplify their calculations.
How were Mayan scribes trained in mathematics?
Mayan scribes and priests likely received specialized education in mathematics, often passed down within elite classes. Training included studying codices, using counting tools, and learning from mentors who were skilled in calendrical and astronomical calculations.
Were Mayan mathematical principles shared with other civilizations?
While there’s no direct evidence of interaction with other ancient civilizations, the Maya developed mathematical principles independently. Their achievements were unique to their culture and region, showcasing their innovative thinking without outside influence.
How did the Spanish conquest affect Mayan mathematical knowledge?
The Spanish conquest led to the destruction of many Mayan codices and records, resulting in the loss of significant knowledge. However, some surviving documents, like the Dresden Codex, have preserved key insights into Mayan mathematical and astronomical skills.

